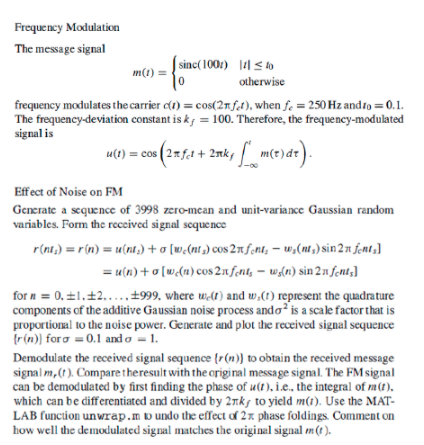
Frequency Modulation The message signal m(1) sinc(1001) 'to 0 otherwise frequency modulates the carrier (1) = cos(21fct), when fo = 250 Hz andto=0.1. The frequency deviation constant is k; = 100. Therefore, the frequency-modulated signal is ) Effect of Noise on FM Generate a sequence of 3998 zero-mcan and unit-variance Gaussian random variables. Form the received signal sequence r(n) =r(n) = u(nt.) + 0 [we(nt) cos2nfent. - w;(ntz) sin 2 fents] = u(n)+ 0 [we(n) cos 2nfant, - Ws(m) sin 2nfent;] for n = 0. +1, +2...., 1999, where welt) and w,(t) represent the quadrature components of the additive Gaussian noise process ando is a scale factor that is proportional to the noise power. Generate and plot the received signal sequence frin)) foro = 0.1 and o = 1. Demodulate the received signal sequence fr(n)) to obtain the received message signalm, (t). Compare theresult with the original message signal. The FM signal can be demodulated by first finding the phase of u(t), i.e., the integral of m(t). which can be differentiated and divided by 2ks to yield m(t). Use the MAT- LAB function unwrap.m wundo the effect of 2x phase foldings Comment on how well the demodulated signal matches the original signal m(t).