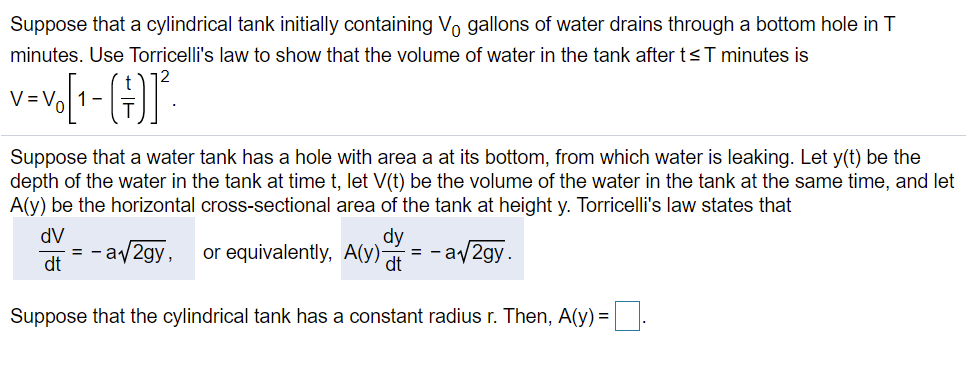
Suppose that a cylindrical tank initially containing Vo gallons of water drains through a bottom hole in T minutes. Use Torricelli's law to show that the volume of water in the tank after t sT minutes is v=vo[-- (47)] Suppose that a water tank has a hole with area a at its bottom, from which water is leaking. Let y(t) be the depth of the water in the tank at time t, let V(t) be the volume of the water in the tank at the same time, and let A(y) be the horizontal cross-sectional area of the tank at height y. Torricelli's law states that dV dy -= -a/2gy, or equivalently, Aly) = -av av2gy. dt dt Suppose that the cylindrical tank has a constant radius r. Then, A(Y) = D.