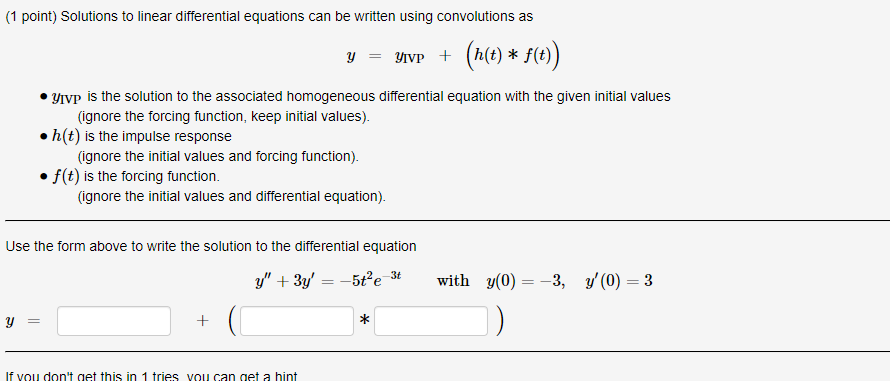
(1 point) Solutions to linear differential equations can be written using convolutions as y = YiVp + (h(t) * f(t)) Yip is the solution to the associated homogeneous differential equation with the given initial values (ignore the forcing function, keep initial values). • h(t) is the impulse response (ignore the initial values and forcing function). f(t) is the forcing function. (ignore the initial values and differential equation). Use the form above to write the solution to the differential equation y" + 3y' = -5t’e 3t with y(0) = -3, y'(0= 3 y + * If you don't get this in 1 tries you can get a hint.