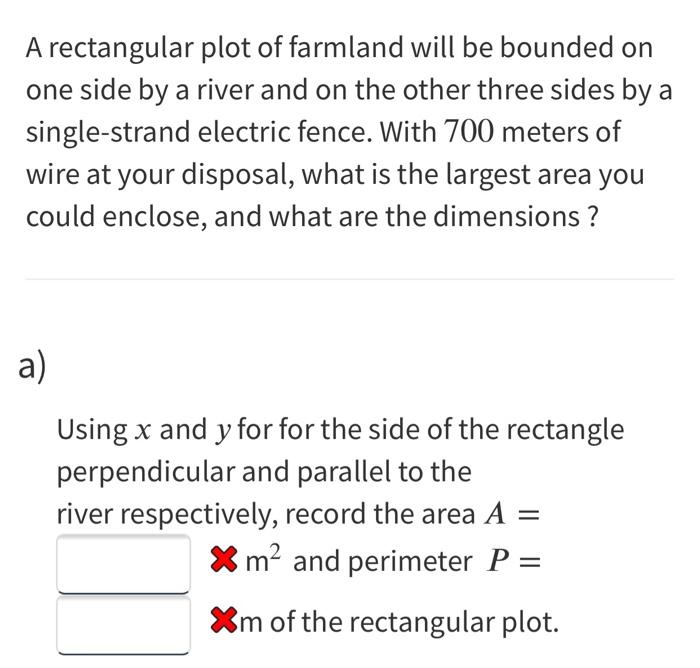
A rectangular plot of farmland will be bounded on one side by a river and on the other three sides by a single-strand electric fence. With 700 meters of wire at your disposal, what is the largest area you could enclose, and what are the dimensions ? a) Using x and y for for the side of the rectangle perpendicular and parallel to the river respectively, record the area A X m2 and perimeter P = Xm of the rectangular plot.
b) Using the fact that only 700 meters of wire are at your disposal express y through x: y = Use above to express area (A) as a function of x only: A =
Now maximise A, finding x max = m and corresponding Amax (use 3 significant figures) In your working remember to use Turning points and then find A" (x) and check that A" (x max) < 0, which ensures Xmax corresponds to local maximum for A(x). Also check that A(x) at x = 0) and y = 0 (edges of the possible range for x and y values) give A = 0, therefore Amax is indeed the maximum possible area.