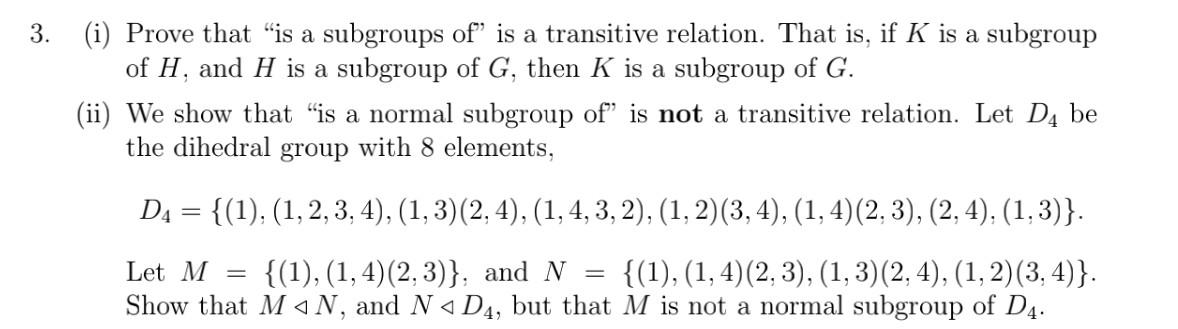
3. (i) Prove that "is a subgroups of' is a transitive relation. That is, if K is a subgroup of H, and H is a subgroup of G, then K is a subgroup of G. (ii) We show that “is a normal subgroup of" is not a transitive relation. Let D4 be the dihedral group with 8 elements, D4 = {(1), (1, 2, 3, 4), (1, 3)(2,4), (1,4,3,2), (1, 2)(3, 4), (1, 4)(2,3), (2, 4), (1,3)}. Let M {(1), (1,4)(2,3)}, and N {(1), (1, 4)(2, 3), (1, 3)(2,4), (1, 2)(3,4)}. Show that M - N, and N - D4, but that M is not a normal subgroup of D4.