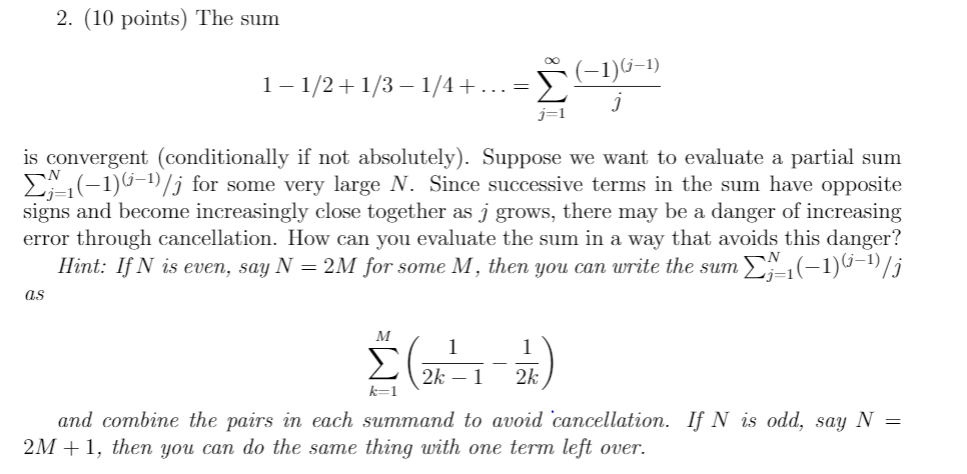
2. (10 points) The sum (-1)6-1) 1-1/2+1/3 – 1/4+...= j=1 N is convergent (conditionally if not absolutely). Suppose we want to evaluate a partial sum (-1)(–1)|j for some very large N. Since successive terms in the sum have opposite signs and become increasingly close together j grows, there may be a danger of increasing error through cancellation. How can you evaluate the sum in a way that avoids this danger? Hint: If N is even, say N = 2M for some M, then you can write the sum (–1(-1)6-1)/j as M Σ 1 2k - 1 1 2k k=1 and combine the pairs in each summand to avoid cancellation. If N is odd, say N = 2M +1, then you can do the same thing with one term left over.