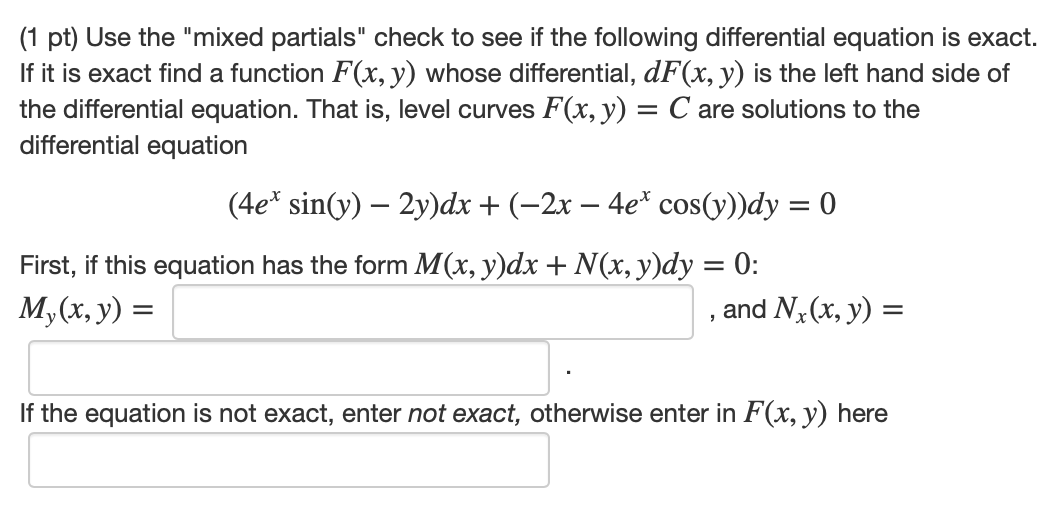
(1 pt) Use the "mixed partials" check to see if the following differential equation is exact. If it is exact find a function F(x, y) whose differential, dF(x, y) is the left hand side of the differential equation. That is, level curves F(x, y) = C are solutions to the differential equation (4e* sin(y) – 2y)dx + (-2x – 4e* cos(y))dy = 0 First, if this equation has the form M(x, y)dx + N(x, y)dy = 0: My(x, y) = and Nx(x, y) = 2 If the equation is not exact, enter not exact, otherwise enter in F(x, y) here