All the solutions posted for this question don't seem correct.Can you please confirm my answers with working! thanks

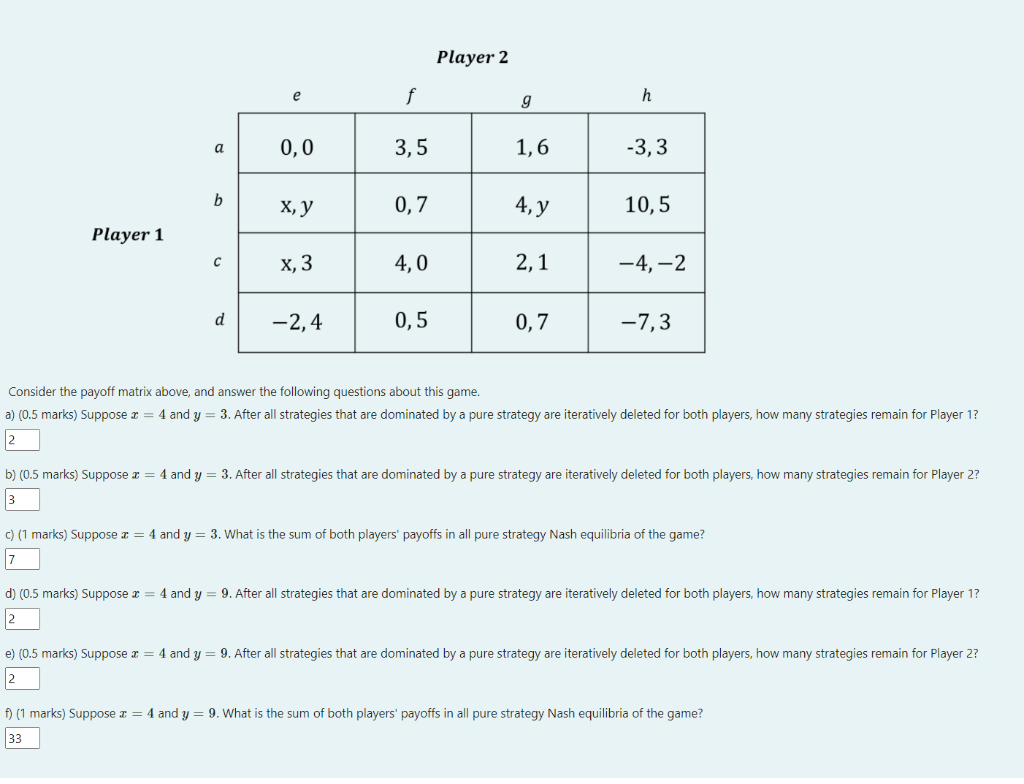
Player 2 e f h g a 0,0 3,5 1,6 -3,3 b x, y 0,7 4, y 10,5 Player 1 с X,3 4,0 2,1 -4,-2 d -2,4 0,5 0,7 -7,3 Consider the payoff matrix above, and answer the following questions about this game. a) (0.5 marks) Suppose x = 4 and y = 3. After all strategies that are dominated by a pure strategy are iteratively deleted for both players, how many strategies remain for Player 1? 2 b) (0.5 marks) Suppose < = 4 and y = 3. After all strategies that are dominated by a pure strategy are iteratively deleted for both players, how many strategies remain for Player 2? c) (1 marks) Suppose I = 4 and y = 3. What is the sum of both players' payoffs in all pure strategy Nash equilibria of the game? 7 d) (0.5 marks) Suppose x = 4 and y = 9. After all strategies that are dominated by a pure strategy are iteratively deleted for both players, how many strategies remain for Player 1? 2 e) (0.5 marks) Suppose x = 4 and y = 9. After all strategies that are dominated by a pure strategy are iteratively deleted for both players, how many strategies remain for Player 2? 2 pure strategy Nash equilibria of the game? f) (1 marks) Suppose = 4 and y = 9. What is the sum of both players' payoffs in 33